Draw the Binary Search Tree That Results

Binary Tree-

Before you go through this article, make sure that you gone through the previous article on Binary Trees.

We have discussed-

• Binary tree is a special tree data structure.
• In a binary tree, each node can have at most 2 children.
• In a binary tree, nodes may be arranged in any random order.

In this article, we will discuss about Binary Search Trees.

Binary Search Tree-

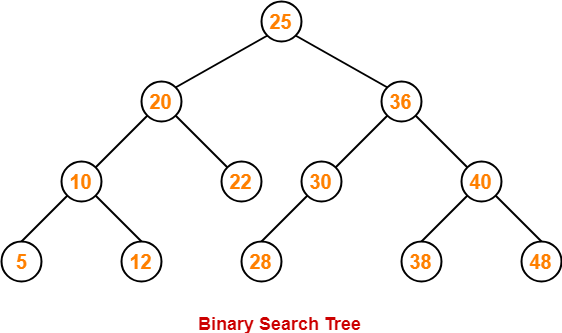
Binary Search Tree is a special kind of binary tree in which nodes are arranged in a specific order.

In a binary search tree (BST), each node contains-

• Only smaller values in its left sub tree
• Only larger values in its right sub tree

Example-

Number of Binary Search Trees-

Example-

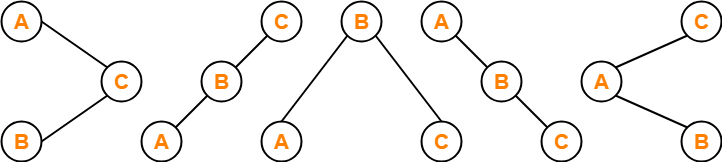
Number of distinct binary search trees possible with 3 distinct keys

= ^2×3C₃ / 3+1

= ⁶C₃ / 4

= 5

If three distinct keys are A, B and C, then 5 distinct binary search trees are-

Binary Search Tree Construction-

Let us understand the construction of a binary search tree using the following example-

Example-

Construct a Binary Search Tree (BST) for the following sequence of numbers-

50, 70, 60, 20, 90, 10, 40, 100

When elements are given in a sequence,

• Always consider the first element as the root node.
• Consider the given elements and insert them in the BST one by one.

The binary search tree will be constructed as explained below-

Insert 50-

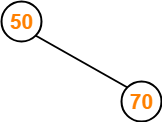
Insert 70-

• As 70 > 50, so insert 70 to the right of 50.

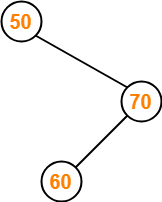
Insert 60-

• As 60 > 50, so insert 60 to the right of 50.
• As 60 < 70, so insert 60 to the left of 70.

Insert 20-

• As 20 < 50, so insert 20 to the left of 50.

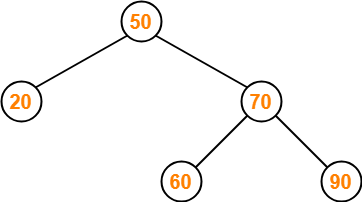
Insert 90-

• As 90 > 50, so insert 90 to the right of 50.
• As 90 > 70, so insert 90 to the right of 70.

Insert 10-

• As 10 < 50, so insert 10 to the left of 50.
• As 10 < 20, so insert 10 to the left of 20.

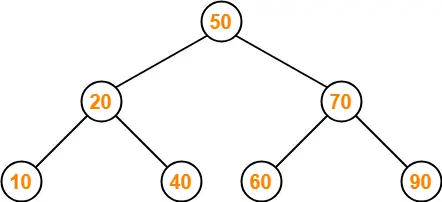
Insert 40-

• As 40 < 50, so insert 40 to the left of 50.
• As 40 > 20, so insert 40 to the right of 20.

Insert 100-

• As 100 > 50, so insert 100 to the right of 50.
• As 100 > 70, so insert 100 to the right of 70.
• As 100 > 90, so insert 100 to the right of 90.

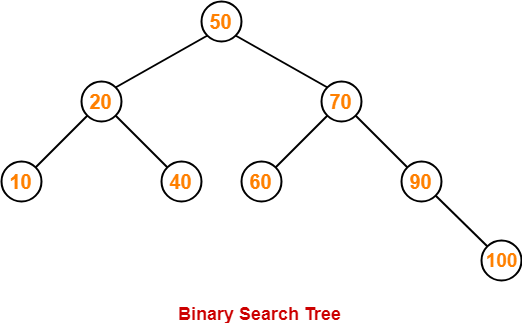
This is the required Binary Search Tree.

To gain better understanding about Binary Search Trees,

Watch this Video Lecture

PRACTICE PROBLEMS BASED ON BINARY SEARCH TREES-

Problem-01:

A binary search tree is generated by inserting in order of the following integers-

50, 15, 62, 5, 20, 58, 91, 3, 8, 37, 60, 24

The number of nodes in the left subtree and right subtree of the root respectively is _____.

1. (4, 7)
2. (7, 4)
3. (8, 3)
4. (3, 8)

Solution-

Using the above discussed steps, we will construct the binary search tree.

The resultant binary search tree will be-

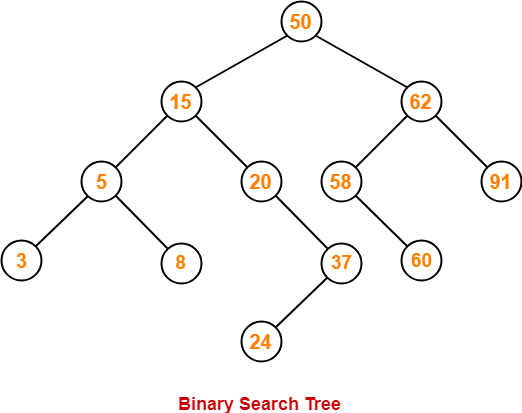
Clearly,

• Number of nodes in the left subtree of the root = 7
• Number of nodes in the right subtree of the root = 4

Thus, Option (B) is correct.

Problem-02:

How many distinct binary search trees can be constructed out of 4 distinct keys?

1. 5
2. 14
3. 24
4. 35

Solution-

Number of distinct binary search trees possible with 4 distinct keys

= ²ⁿC_n / n+1

= ^2×4C₄ / 4+1

= ⁸C₄ / 5

= 14

Thus, Option (B) is correct.

Problem-03:

The numbers 1, 2, …, n are inserted in a binary search tree in some order. In the resulting tree, the right subtree of the root contains p nodes. The first number to be inserted in the tree must be-

1. p
2. p+1
3. n-p
4. n-p+1

Solution-

Let n = 4 and p = 3.

Then, given options reduce to-

1. 3
2. 4
3. 1
4. 2

Our binary search tree will be as shown-

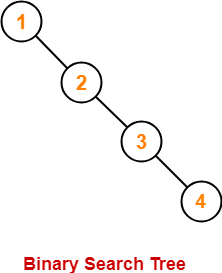
Clearly, first inserted number = 1.

Thus, Option (C) is correct.

Problem-04:

We are given a set of n distinct elements and an unlabeled binary tree with n nodes. In how many ways can we populate the tree with given set so that it becomes a binary search tree?

1. 0
2. 1
3. n!
4. C(2n, n) / n+1

Solution-

Option (B) is correct.

To watch video solutions and practice more problems,

Watch this Video Lecture

Download Handwritten Notes Here-

Next Article- Binary Search Tree Traversal

Get more notes and other study material of Data Structures.

Watch video lectures by visiting our YouTube channel LearnVidFun.

Summary

Article Name

Binary Search Tree | Example | Construction

Description

Binary Search Tree or BST is a special kind of binary tree. Binary Search Tree Example is given. Binary Search Tree Construction- Various steps to construct binary search tree are given. Practice Problems on Binary Search Trees.

Author

Akshay Singhal

Publisher Name

Gate Vidyalay

Publisher Logo

wrennboodgme.blogspot.com

Source: https://www.gatevidyalay.com/binary-search-trees-data-structures/

Share this post